
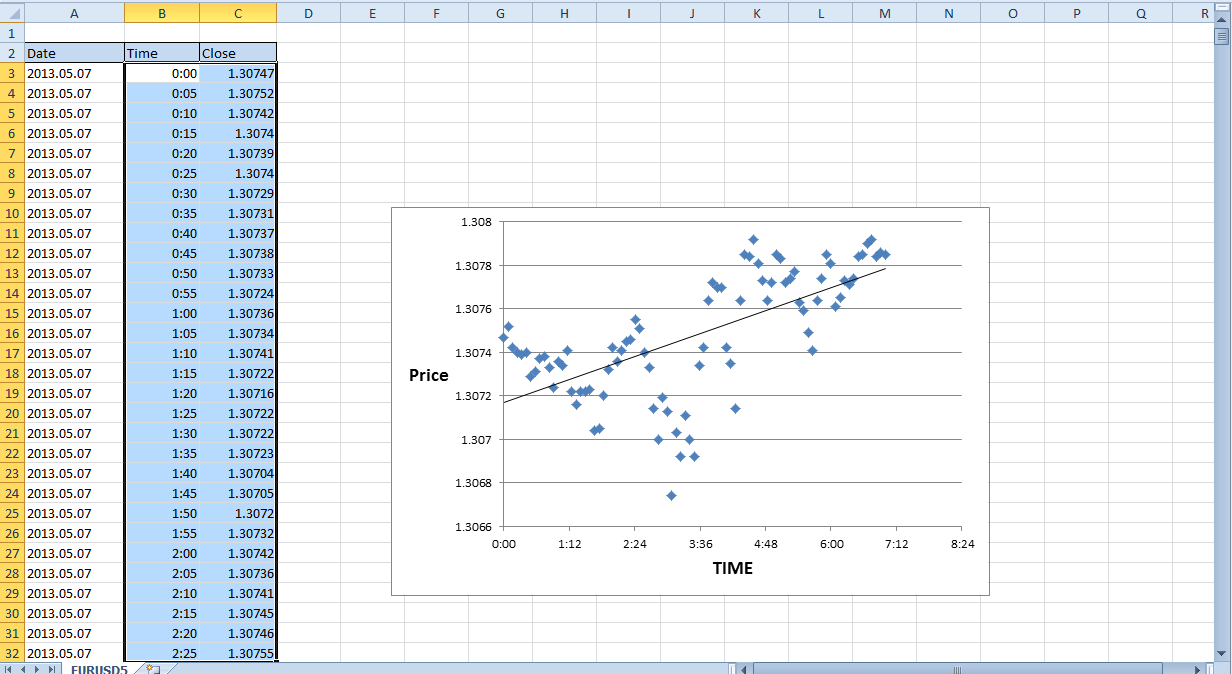
The values for m and b (slope and intercept, respectively) are calculated from the data using the simple linear regression. The calculation of the line is a little more complex. In this specific case, the equation for the line is y=217.1x-432680 or # Specimens = 217.1*year-432680. The basis of this line is the equation we all learned in high school, which is y=mx+b, where y = # of specimens and x is the year. This line is a model that comes from the simple linear regression of the data. Many have questioned how this line is calculated and what it means. In Microsoft Excel, this can be done by inserting a trendline. These results in table form are… YearĪ simple scatter plot places a dot where each year intersects the number of specimens collected in that year.Īnother useful feature of scatter plots is that they are easily completed by simple linear regression in the placement of a regression line through the data. In the picture, the number of specimens recovered by year is depicted.
Below is a photo of Pit 91 along with the data I chose to use to introduce a scatter plot. On my trip to La Brea, the scientists and researchers were actively working in Pit 91. Most of the current activity and excavation revolve around Pit 91. Since the 1940s, the pits have been excavated resulting in the discovery of many fossils, including Mammoths, Wolf, Bear, and some other lesser known animals such as the American Lion and American Camel. These pits have existed for thousands of years, forming a natural trap contributing to the early demise of many an animal unlucky enough to stray into the tar. The La Brea Tar Pits, located in Los Angeles, are a natural formation where tar seeps to the surface. To illustrate this, I collected some data on a recent trip to the La Brea Tar Pits.

It is often combined with a simple linear regression line used to fit a model between the two variables.


 0 kommentar(er)
0 kommentar(er)
